

Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables.
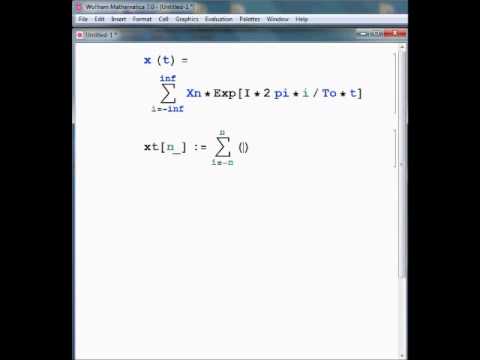
Exponential integral of imaginary argument 2.8 Exponential integral of imaginary argumentįor real non-zero values of x, the exponential integral Ei( x) is defined asĮi ( x ) = − ∫ − x ∞ e − t t d t = ∫ − ∞ x e t t d t.2.3 Exponential and logarithmic behavior: bracketing.See either fedja's Banach space argument, or my sketchier iteration argument. If we want $f(f(z)) = e^z+z-1$, then there will be a solution, analytic in a neighborhood of the real axis. Let me see if I can summarize the conversation so far. Smoothness in Ecalle's method for fractional iterates.Rational functions with a common iterate.The functional equation $f(f(x))=x+f(x)^2$.The non-convergence of f(f(x))=exp(x)-1 and labeled rooted trees.Closed form functions with half-exponential growth.Does the exponential function has a square root.What can be said about f(x) and about other functions with such an intermediate growth behavior? Can such an intermediate growth behavior be represented by analytic functions? Is this function f(x) or other functions with such an intermediate growth relevant to any interesting mathematics? (It appears that quite a few interesting mathematicians and other scientists thought about this function/growth-rate.) On the other hand the function f is subexponential (even in the CS sense f(x)=exp (o(x))), subsubexponential (f(x)=exp exp (o(log x))) subsubsub exponential and so on. The history of PDEs goes back to the works of famous eighteenth-century mathematicians such as Euler, d’Alembert. The growth rate of the function f (as x goes to infinity) is larger than linear (linear means O(x)), polynomial (meaning exp (O(log x))), quasi-polynomial (meaning exp(exp O(log log x))) quasi-quasi-polynomial etc. My aim in writing this post is to give you a brief glimpse into the fascinating world of PDEs using the improvements for boundary value problems in DSolve and the new DEigensystem function in Version 10.3 of the Wolfram Language. The question is open ended and it was discussed quite recently in the comment thread in Aaronson's blog here
#Exponential in mathematica pdf
In addition, the tails of the PDF are 'thin', in the sense that the PDF decreases exponentially for large values of. defining integral indeed gives Gamma function In789: IntegratexHp-1L Exp-xD, 8x. The probability density function (PDF) of an exponential distribution is monotonically decreasing. The above are strictly numeric functions, but Mathematicas Gamma. If you want to simplify these, you need to provide an assumption on the domain of a, e.g., Simplify Log Ea, a Reals a or Simplify Log Ea, a > 0 a as suggested in the other answer. If At is n n, the matrix exponential is defined by eAt exp At I At 12A2t2 n0 1nAntn. Let us first consider the function f(x)2x.

Such a function is called an exponential function.

The question is about the function f(x) so that f(f(x))=exp (x)-1. ExponentialDistribution represents a continuous statistical distribution defined over the interval and parametrized by a positive real number. Since Log is a multi-valued inverse function of Exp, Mathematica doesnt evaluate Log Exp a (or equivalently, Log Ea ). Now we want to look at functions where x is in the exponent.


 0 kommentar(er)
0 kommentar(er)
